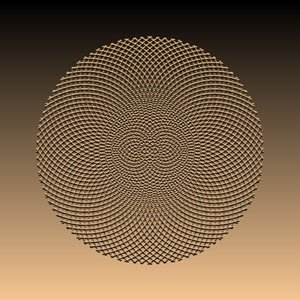
Point patterns are an interesting method to represent a variety of mathematical based forms. They are capable of displaying properties of surface, direction, and line without being any of those, literally, the viewer connects the dots.  This series explores Fermat's Spiral as a method to create radial point figures.

 This spiral, if represented as seeds can be seen in daises, sunflowers, pineapples, and pinecones. A variety of patterns can be created by the Fermat's Spiral based on different angle intervals and number of points that control the scale of the figure and the packing of the spiral.  A set of eight spirals are combined to form a mandala and then rendered.  When combined, an entirely new set of intricate patterns emerge, all different, depending on the number and size of the placed seeds.  The relationship to mandalas is based on the circular form these figures take on, using the simple geometric definition of "mandala"; from the Sanskrit for circle.  A mandala is a complex circular design, intended to draw the eye inward to its center having symmetrical and radial balance.  The Fermat's Spiral in particular is a natural basis for this inward draw.

Check the amazing rich texture and detail.